AFRICA MATHEMATICS ANNALS

Simulation of the blow-up and the quenching time for a positive solutions of
singular boundary value problems for a nonlinear parabolic systems

Halima Nachid⁽¹⁾, Benjamin Yekre ⁽²⁾ and, Yoro Gozo ^(3)
(1) International University of Grand-Bassam, BP 564 Grand-Bassam, CÔTE d'IVOIRE
et Labo. de Modélisation Mathématique et de Calcul Économique LM2CE settat, MAROC
^{(1) (2) (3)} Laboratoire de Mathématiques et Informatique, UFR-SFA,
Université Nangui Abrogoua, 02 BP 801 Abidjan 02, CÔTE d'IVOIRE

Mathematics Subject Classification: (MSC 2010) 35K55, 35B40, 65M06
Key words : Nonlinear parabolic systems, blow-up, parabolic equations, extinction, numerical blow-up time, numerical quenching time, existence, finite difference method. 

Abstract:

We consider the following initial-boundary value problem.

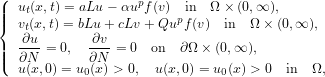
where Ω is a bounded domain in ℝ^N with smooth boundary ∂Ω, L is an elliptic operator, p > 0, α≠0, a,b,c,Q are positive constants, f(s) is a positive and increasing function for the positive values of s.

We find some conditions under which solutions of the above system either exists globally or blow up in a finite time. We also prove that if 0 < p < 1, the solutions u,v) extincts in a finite time. Some numerical results are given to illustrate our analysis.